
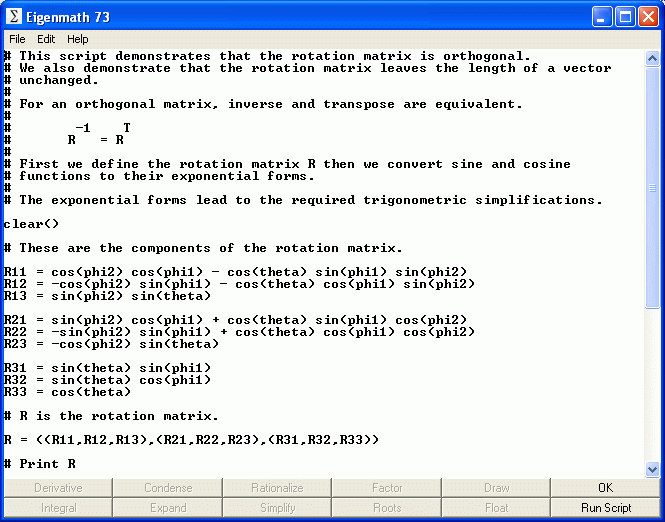
Its eigenfunctions form a basis of the function space on which the operator is defined Īs a consequence, in many important cases, the eigenfunctions of the Hermitian operator form an orthonormal basis.įor many Hermitian operators, notably Sturm–Liouville operators, a third property is Shop your favorite products and we’ll find the best deal with a single click. Eigenmath 2.
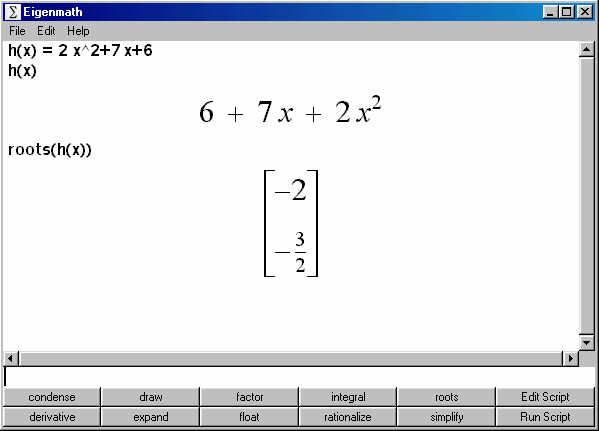
#EIGENMATH FRAC FREE#
Depending on whether the spectrum is discrete or continuous, the eigenfunctions can be normalized by setting the inner product of the eigenfunctions equal to either a Kronecker delta or a Dirac delta function, respectively. Eigenmath free download, and many more programs. Choose Built Products (the default) then click Next. Xcode starts building Eigenmath, which takes some time. For degenerate eigenfunctions with the same eigenvalue λ i, orthogonal eigenfunctions can always be chosen that span the eigenspace associated with λ i, for example by using the Gram-Schmidt process. Launch Xcode and open the project eigenmath-master/xcode/Eigenmath.xcodeproj From the Xcode Product menu, select Archive. The second condition always holds for λ i ≠ λ j. You can reuse the old renderer if you want, because I dont need fx-CG 50 support. Yes, I am working on Eigenmath, so it would be for the fx-9860. For example, consider the derivative operator d d t if i ≠ j Exactly, I dont need support for fx-CG 50. It is based on a keyboard-oriented structure that allows you to write a mathematical expression without any tool. Derivative example Ī widely used class of linear operators acting on infinite dimensional spaces are differential operators on the space C ∞ of infinitely differentiable real or complex functions of a real or complex argument t. Introduction Eigenmath is a graphical application developed with Mathematica that aims to help math students solve complicated mathematical expressions. If multiple linearly independent eigenfunctions have the same eigenvalue, the eigenvalue is said to be degenerate and the maximum number of linearly independent eigenfunctions associated with the same eigenvalue is the eigenvalue's degree of degeneracy or geometric multiplicity. Įach value of λ corresponds to one or more eigenfunctions. The set of all possible eigenvalues of D is sometimes called its spectrum, which may be discrete, continuous, or a combination of both.

Because of the boundary conditions, the possible values of λ are generally limited, for example to a discrete set λ 1, λ 2, … or to a continuous set over some range. The solutions to Equation ( 1) may also be subject to boundary conditions.


 0 kommentar(er)
0 kommentar(er)
